The central aim of our research is to gain knowledge about physical phenomena which occur in condensed matter systems. To achieve this we rely on the tools of many-body physics. Currently, we are investigating whether conducting states of the topological kind exist at an interface between an ideal conductor and an insulator. We are also developing a variational Monte Carlo technique for lattice models, which we are applying to the bosonic Hubbard models in various dimensions. Another ongoing project is the description of transport (conduction, superconductivity, and superfluidity) based on the total momentum and related quantities. We are also investigating generalizations of the magnetic translation group.


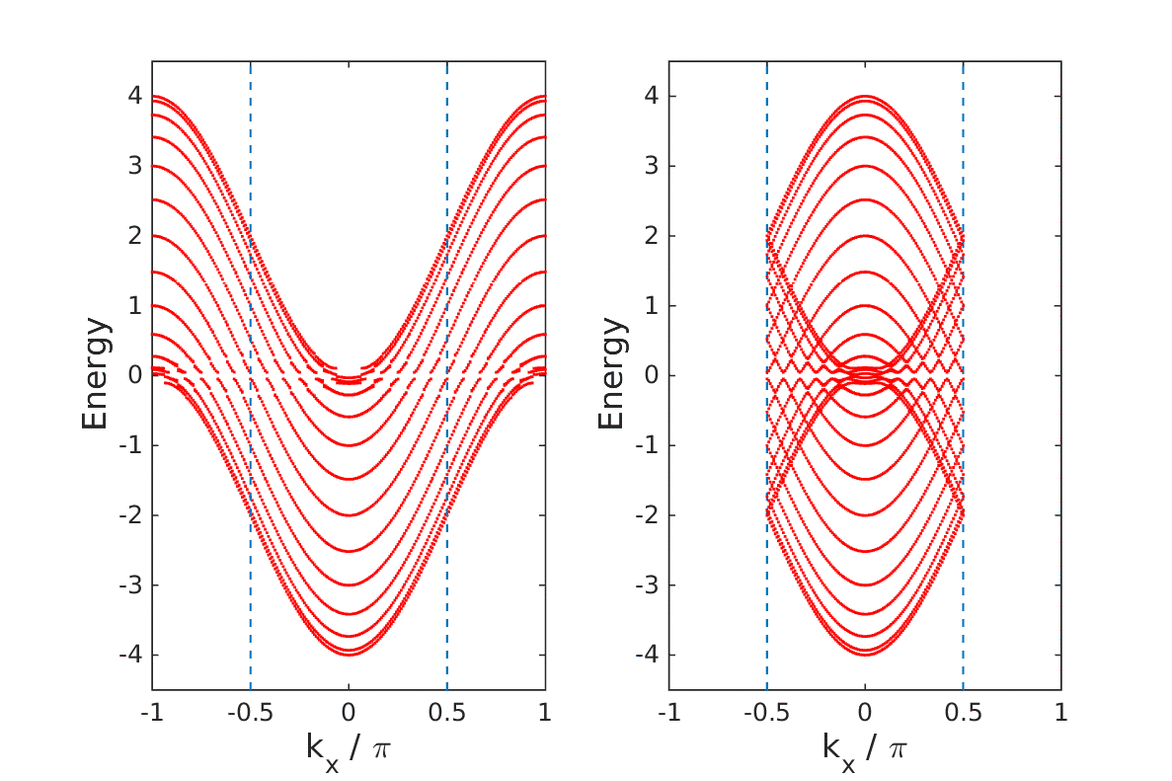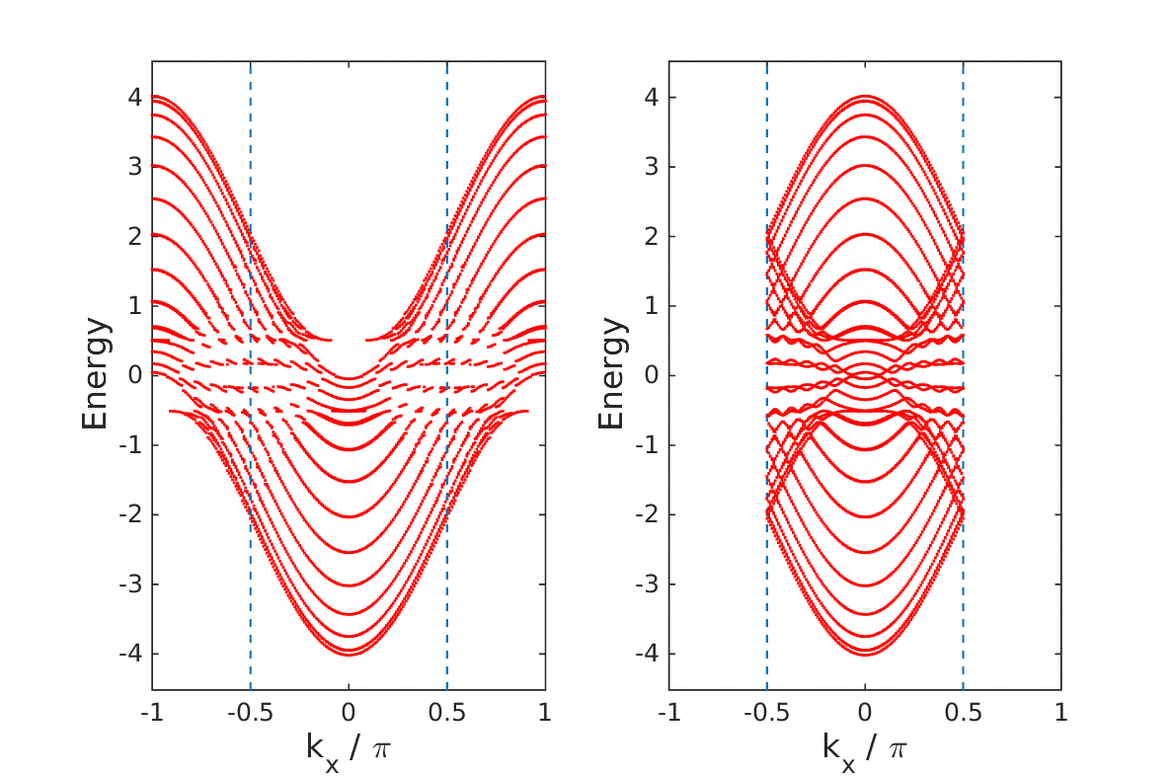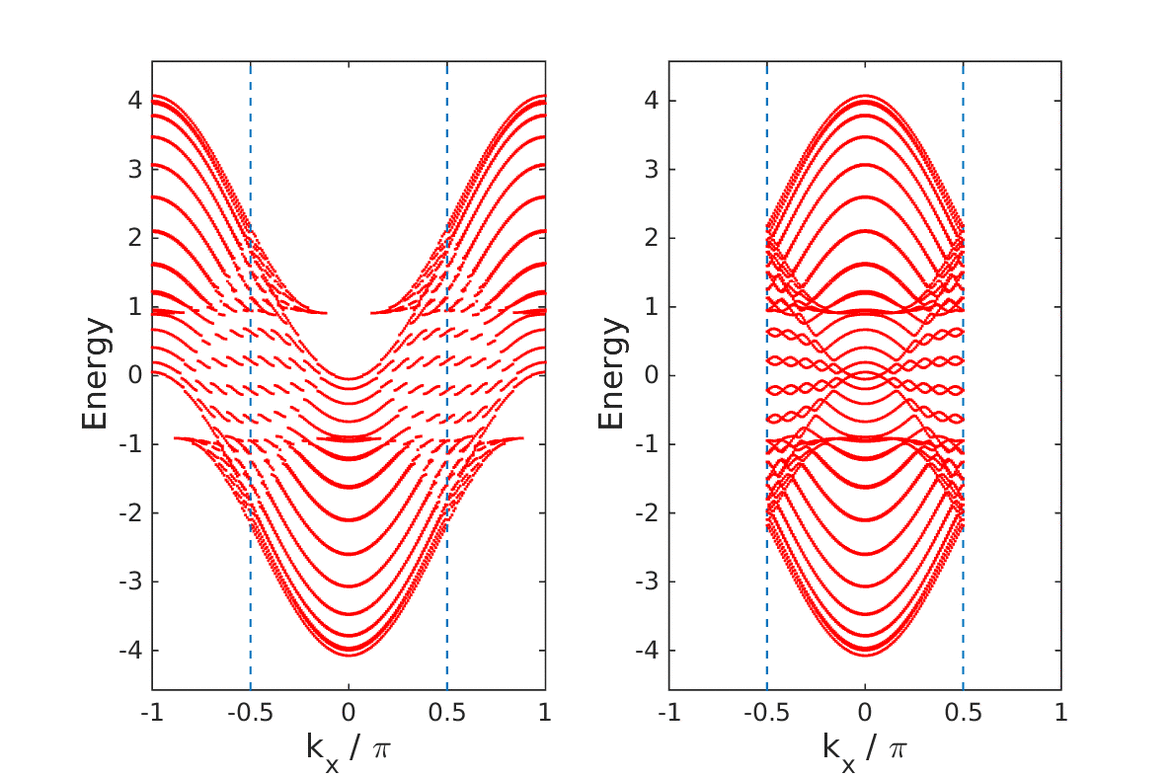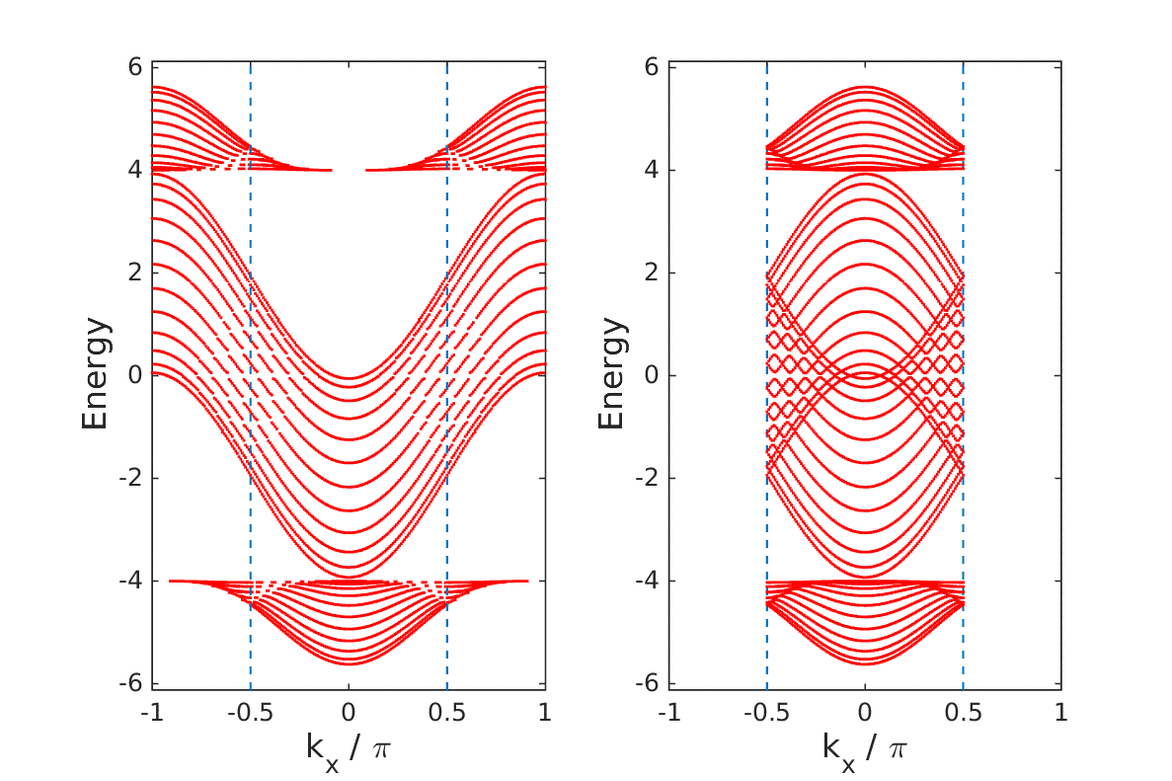
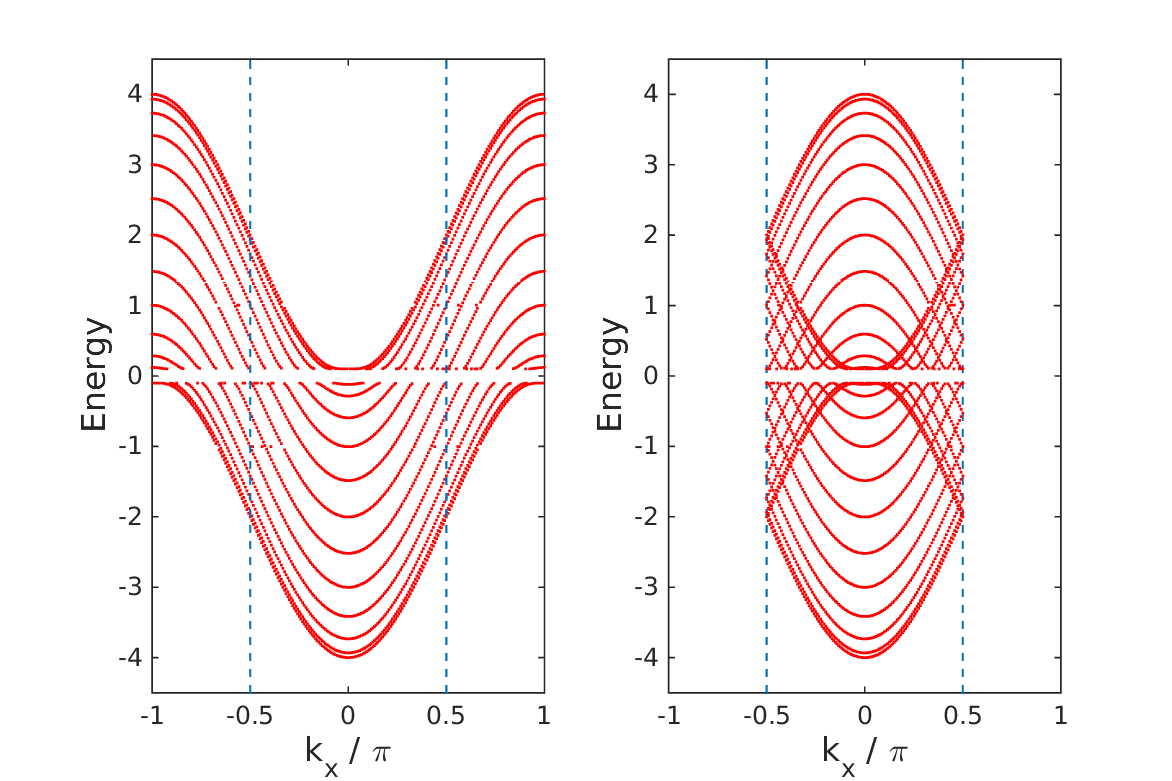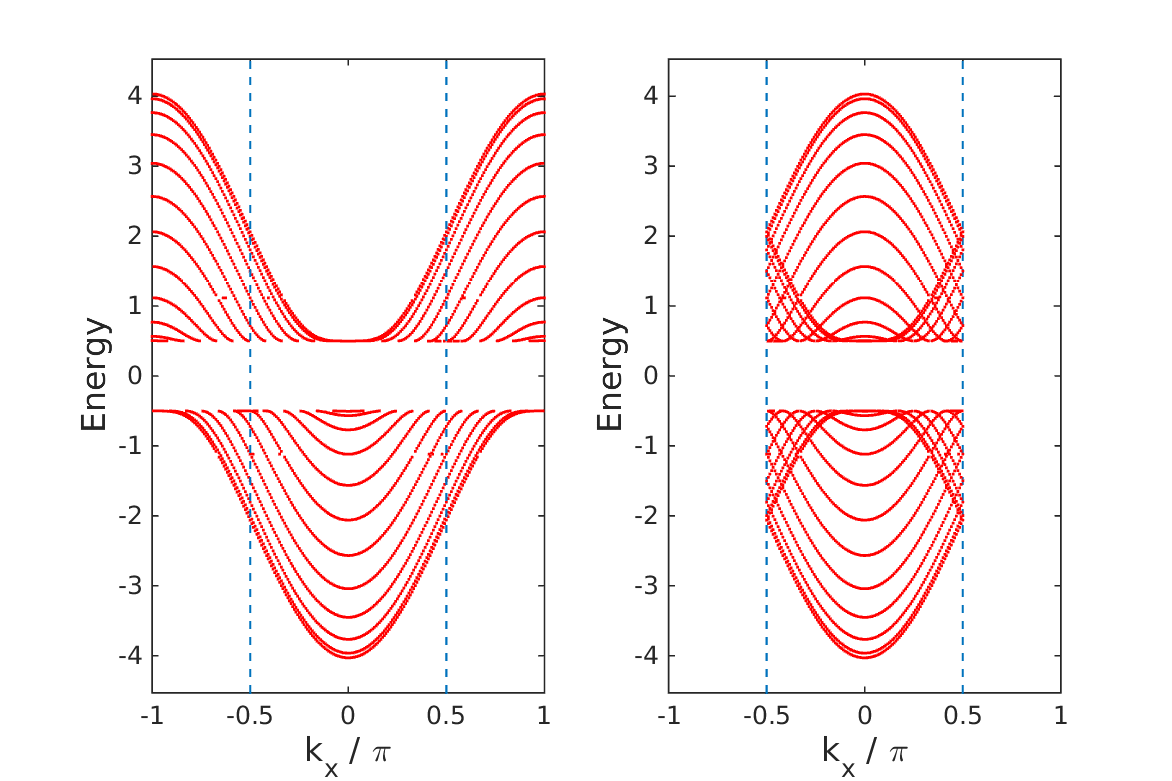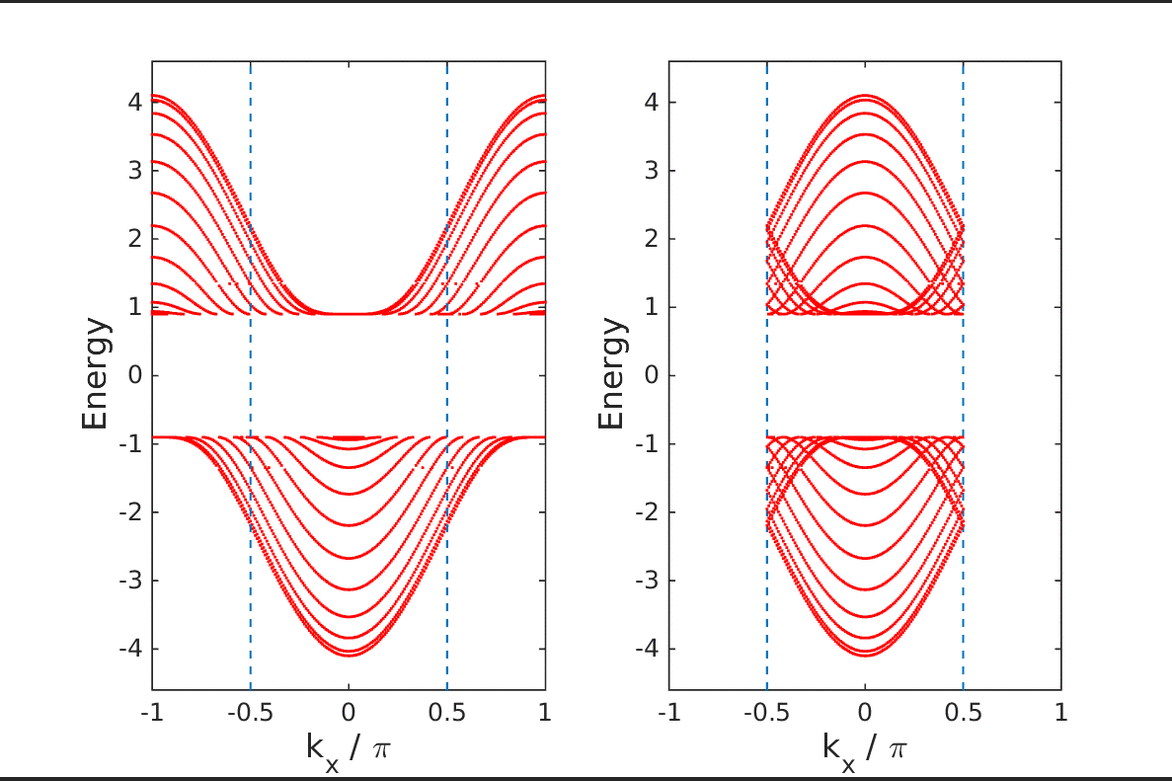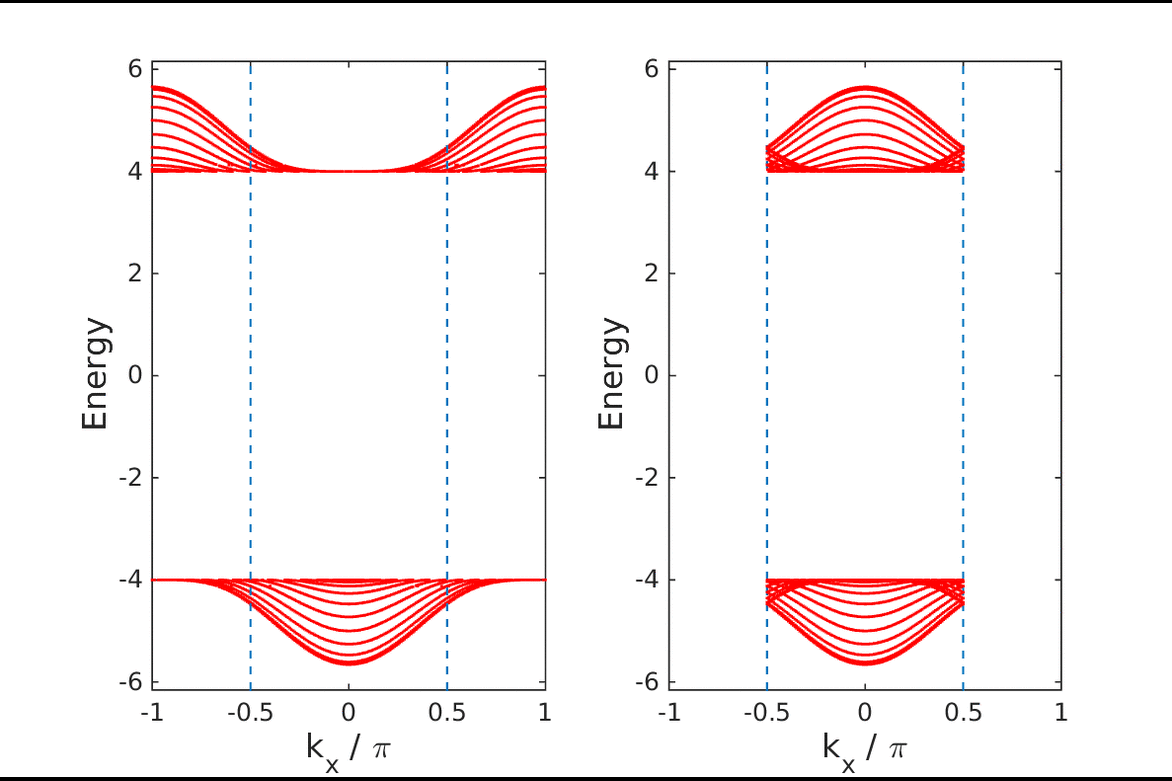
It was recently shown that the transport coefficient of ideal conduction, the Drude weight, can be expressed in terms of a topological invariant. This suggests that an interface between an ideal conductor and an insulator, across which the topological invariant abruptly changes its value, should exhibit topological edge states. We consider the edge at the interface of a simple tight-binding model and a band insulator. We find that crossings in the band structure (one dimensional Dirac points) appear when an interface is present in the system. We calculate the hopping energy along lines of bonds parallel to the interface as a function of distance from the interface. Similarly, we introduce a transport coefficient (Drude weight) which for charge currents running parallel to the interface. We find that charge mobility (both the kinetic energy and the Drude weight) is significantly enhanced in the tight-binding model near the interface.


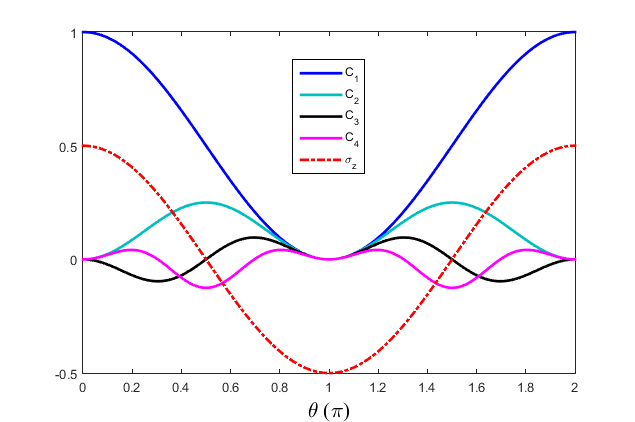
An expansion, similar to the cumulant expansion in probability theory, is carried out for the Bargmann invariant, which is the quantity from which the Berry phase can be derived. The derivation shows that the first term in the expansion corresponds to the Berry phase itself, the higher order terms can be interpreted as the associated cumulants; spread, skew, kurtosis, etc. The gauge invariance of all of these quantities is also demonstrated.


Cumulants of a spin-1/2 particle in a precessing field


  • $C_1= -i \int d \chi \gamma_1$

  • $C_2= - \int d \chi [\gamma_2 - \gamma_2^2] $

  • $C_3= i \int d \chi [\gamma_3 - 3\gamma_2\gamma_1+2\gamma_1^3] $

  • $C_4= \int d \chi [\gamma_4 - 3\gamma_2^2 - 4 \gamma_3\gamma_1 + 12\gamma_1^2\gamma_2 - 6\gamma_1^4] $