Mohammad Yahyavi's personal page


Position: PhD Student

Research Areas: Condensed Matter Physics, Quantum Mechanics, Statistical Mechanics, Computational Materials Science, Disordered systems, Molecular Dynamics Simulation and Nonlinear Dynamics.


Research Projects:


An expansion, similar to the cumulant expansion in probability theory, is carried out for the Bargmann invariant, which is the quantity from which the Berry phase can be derived. The derivation shows that the first term in the expansion corresponds to the Berry phase itself, the higher order terms can be interpreted as the associated cumulants; spread, skew, kurtosis, etc. The gauge invariance of all of these quantities is also demonstrated.


Cumulants of a spin-1/2 particle in a precessing field


  • $C_1= -i \int d \chi \gamma_1$

  • $C_2= - \int d \chi [\gamma_2 - \gamma_2^2] $

  • $C_3= i \int d \chi [\gamma_3 - 3\gamma_2\gamma_1+2\gamma_1^3] $

  • $C_4= \int d \chi [\gamma_4 - 3\gamma_2^2 - 4 \gamma_3\gamma_1 + 12\gamma_1^2\gamma_2 - 6\gamma_1^4] $

It was recently shown that the transport coefficient of ideal conduction, the Drude weight, can be expressed in terms of a topological invariant. This suggests that an interface between an ideal conductor and an insulator, across which the topological invariant abruptly changes its value, should exhibit topological edge states. We consider the edge at the interface of a simple tight-binding model and a band insulator. We find that crossings in the band structure (one dimensional Dirac points) appear when an interface is present in the system. We calculate the hopping energy along lines of bonds parallel to the interface as a function of distance from the interface. Similarly, we introduce a transport coefficient (Drude weight) which for charge currents running parallel to the interface. We find that charge mobility (both the kinetic energy and the Drude weight) is significantly enhanced in the tight-binding model near the interface.

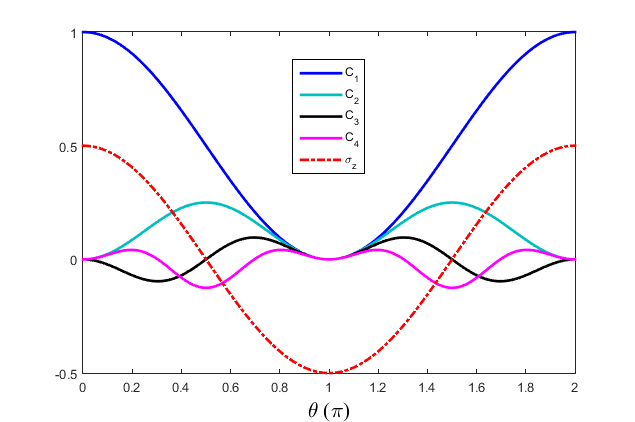
2015 poster

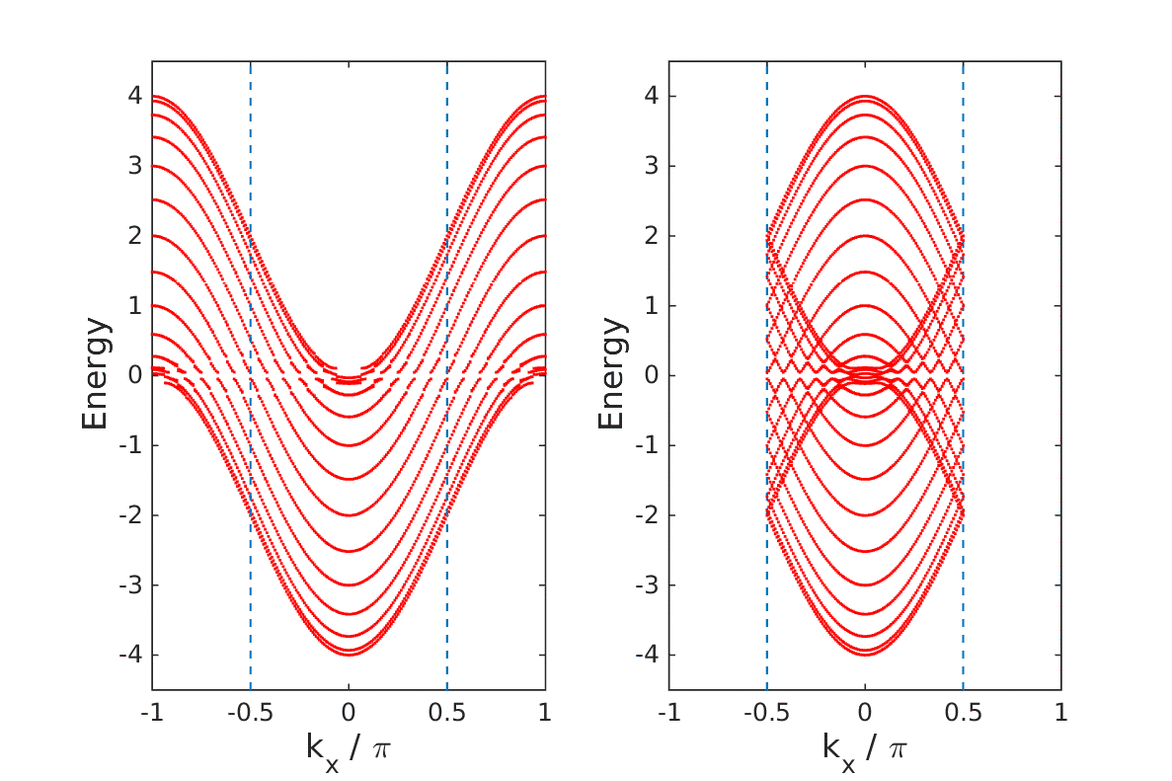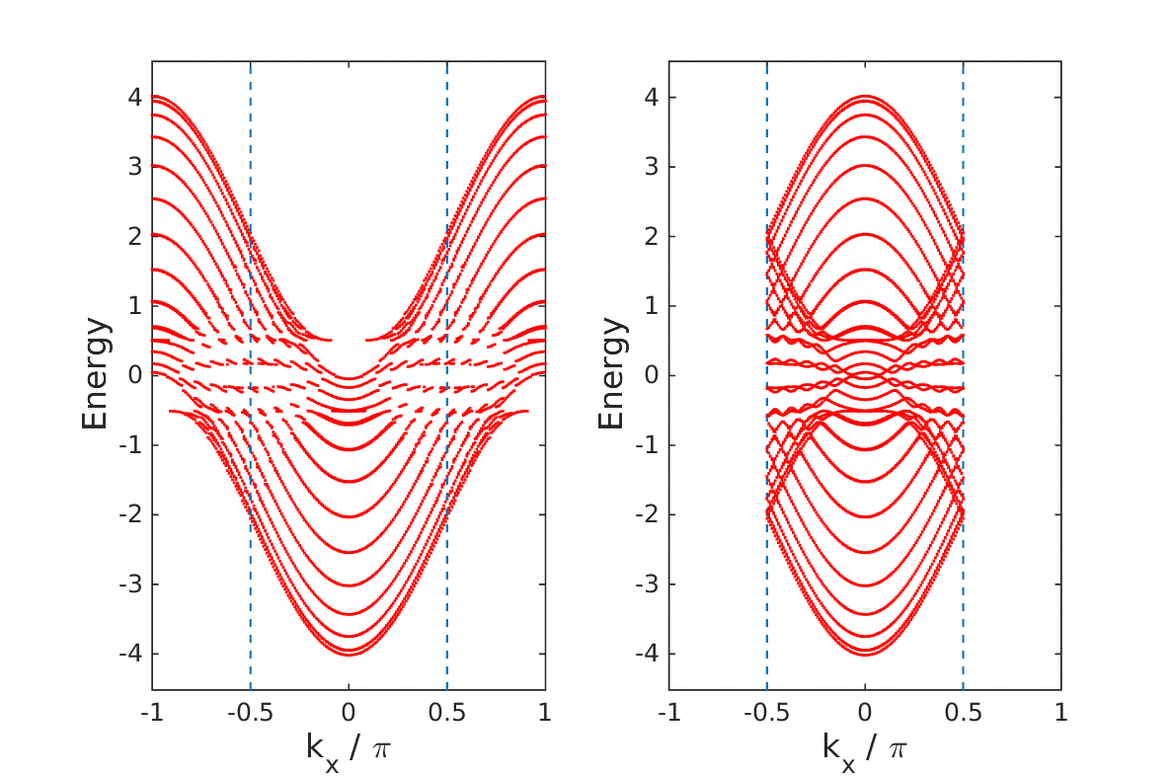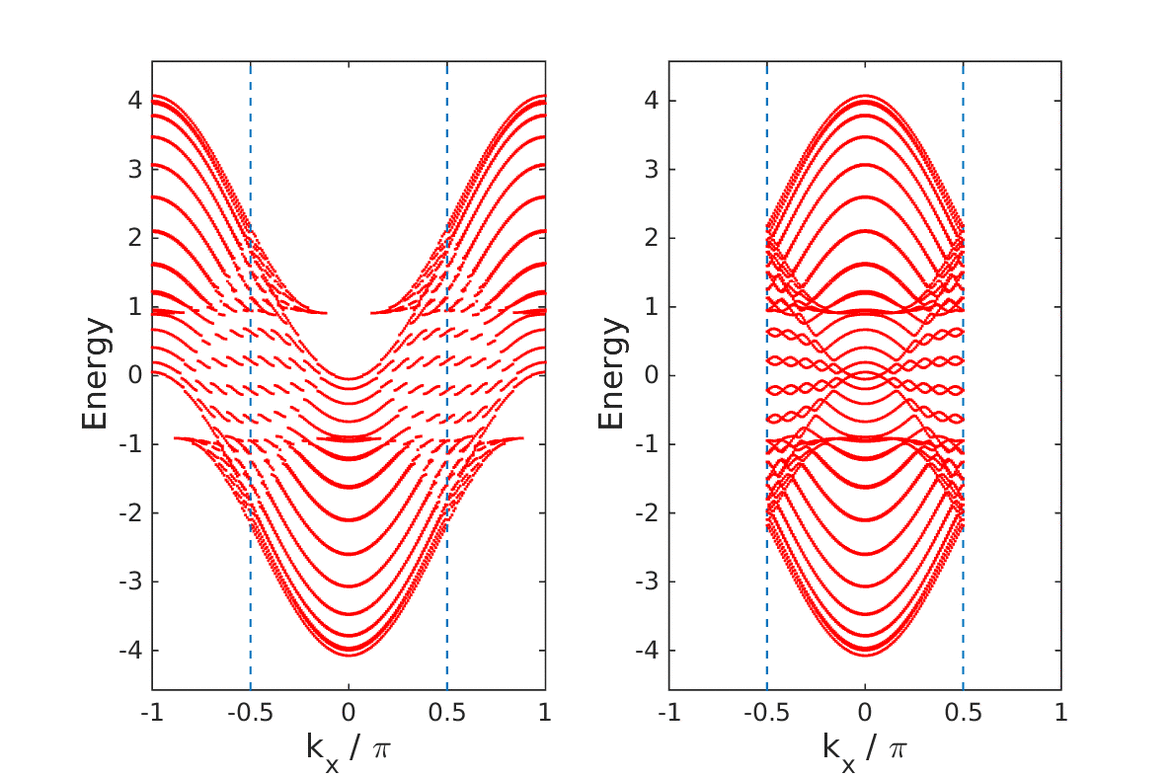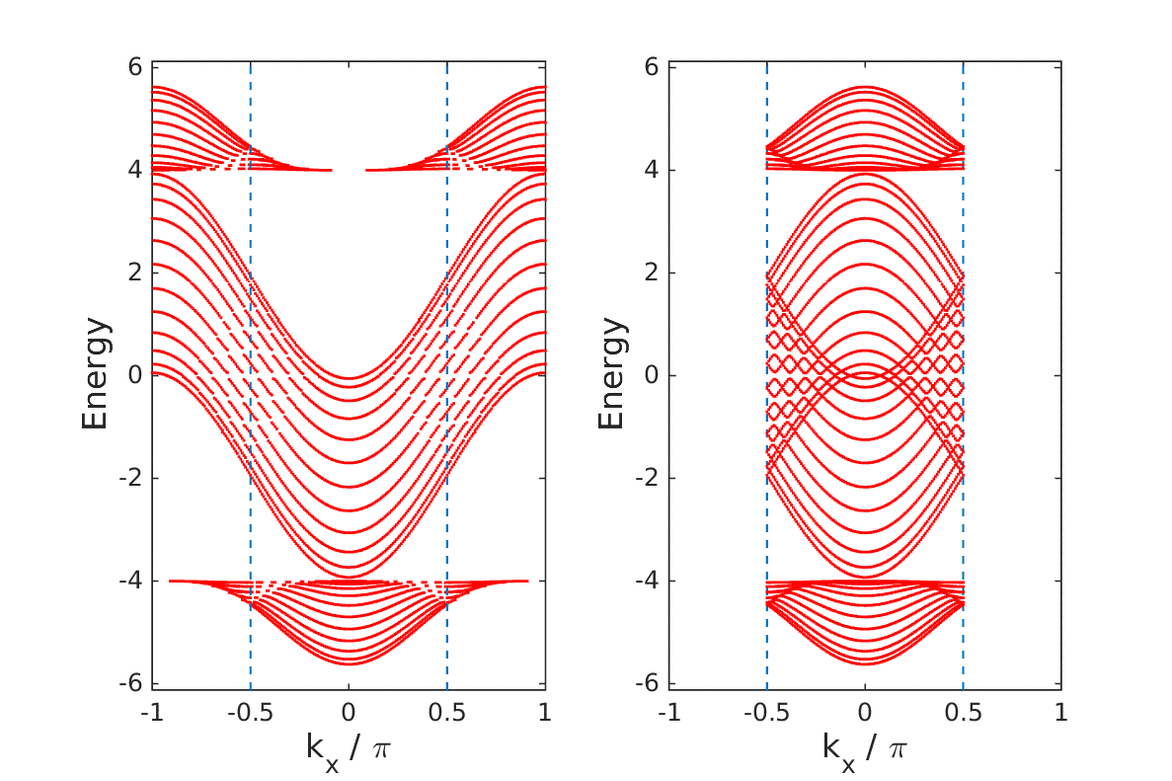
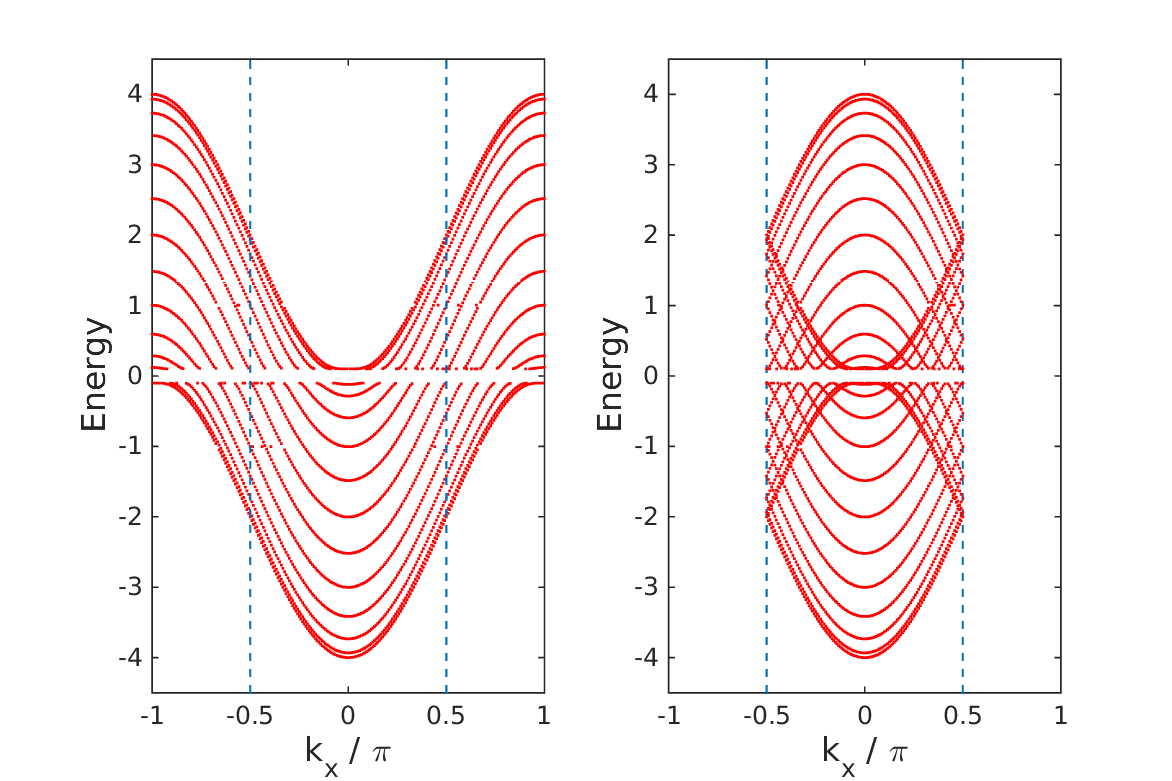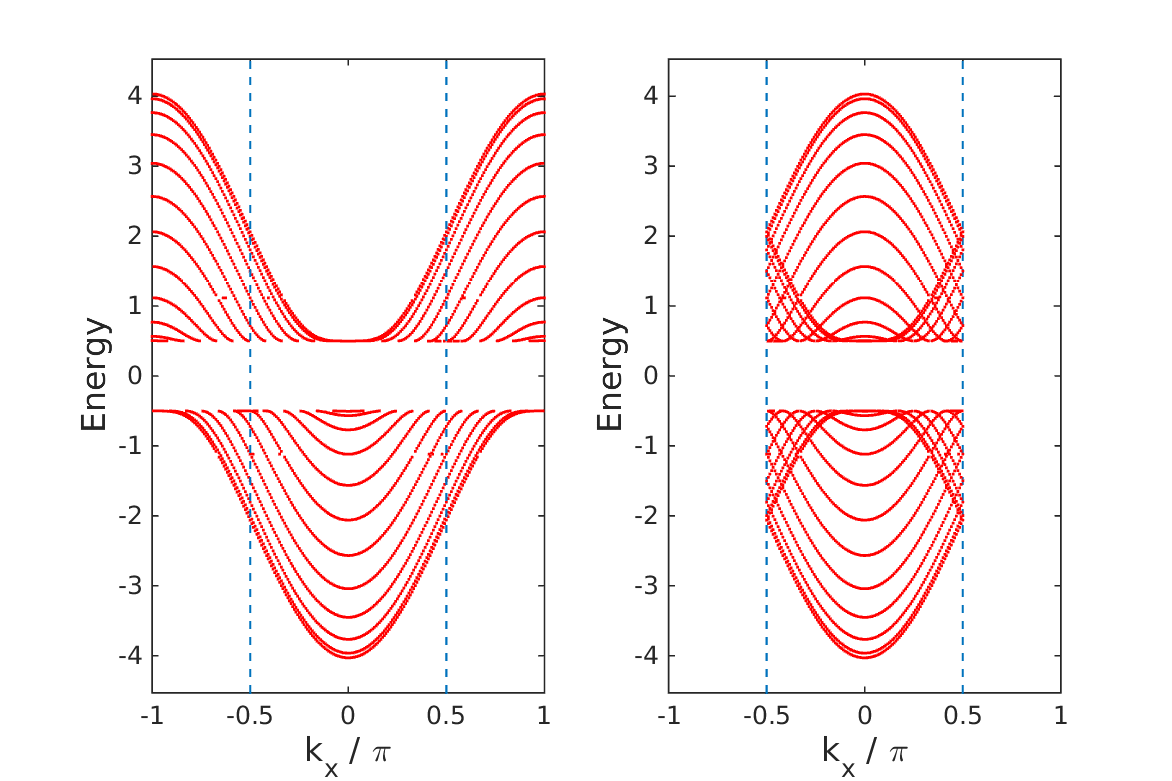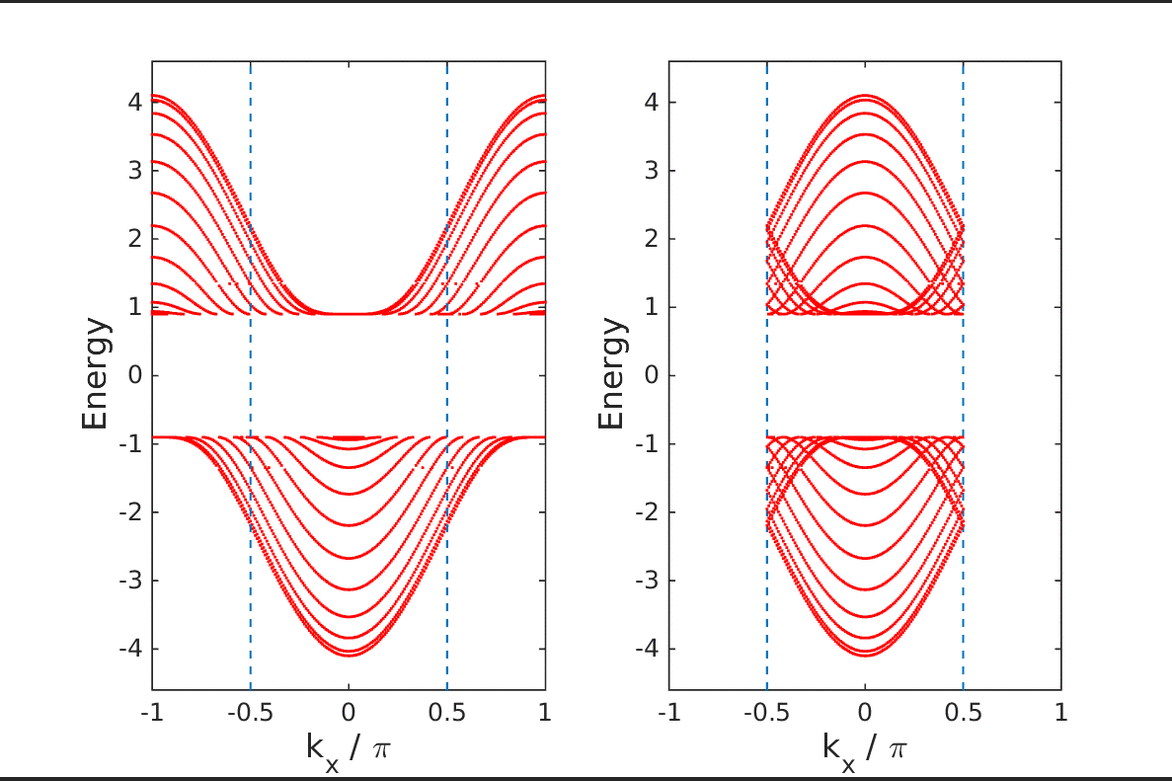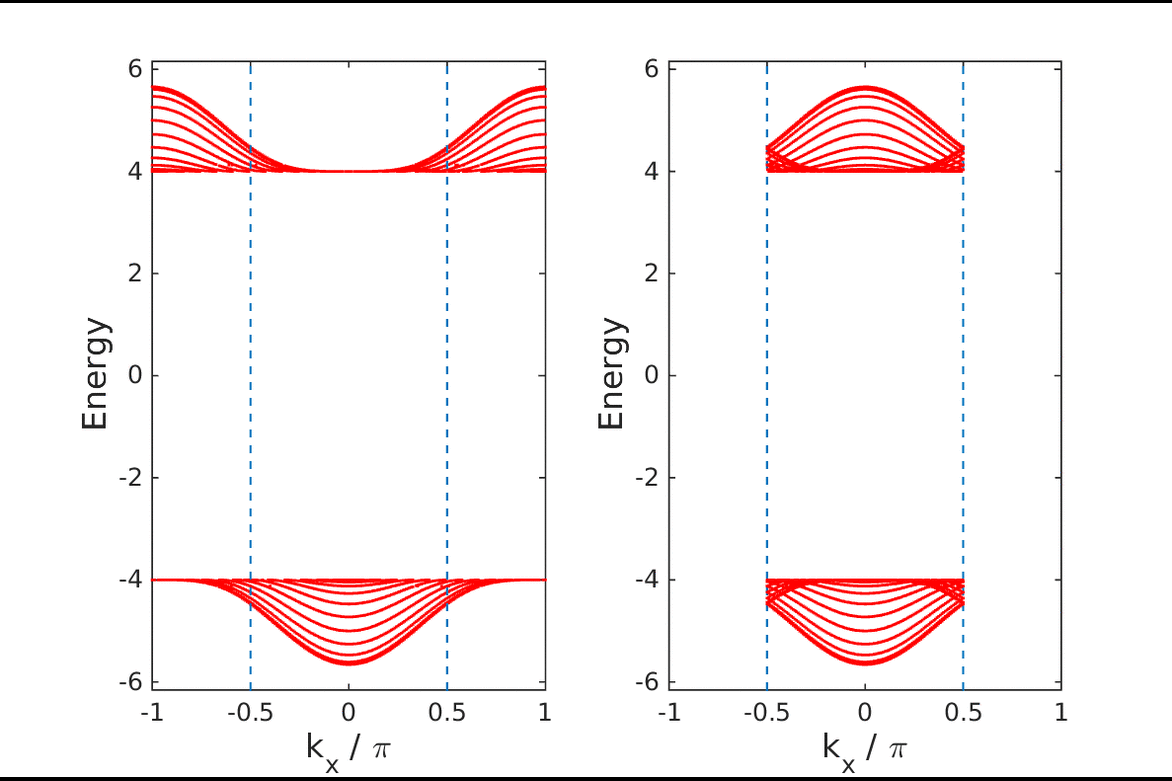
Topological aspects of ideal conduction (pdf) [poster]


Selected Publications:

Enhanced charge transport at the ideal conductor-insulator interface
M. Yahyavi , Balázs Hetényi
----.

Cumulants associated with geometric phases
B. Hetényi, M. Yahyavi
EPL (Europhysics Letters) 105 (4), 40005.

Effect of magnetic field on the radial pulsations of a gas bubble in a non-Newtonian fluid
S. Behnia, F. Mobadersani, M. Yahyavi, A. Rezavand, N. Hoesinpour, A. Ezzat
Chaos, Solitons & Fractals 78, 194-204.

Intelligent controlling microbubble radial oscillations by using Slave–Master Feedback control
S. Behnia, M. Yahyavi, F. Mobadersani
Applied Mathematics and Computation 245, 404-415.

Chaotic behavior of gas bubble in non-Newtonian fluid: a numerical study
S. Behnia, F. Mobadersani, M. Yahyavi, A. Rezavand
Nonlinear Dynamics 74 (3), 559-570.

Observations on the dynamics of bubble cluster in an ultrasonic field
S. Behnia, H. Zahir, M. Yahyavi, A. Barzegar, F. Mobadersani
Nonlinear Dynamics 72 (3), 561-574.

Characterization of intermittency in hierarchy of chaotic maps with invariant measure
S. Behnia, M. Yahyavi
Journal of the Physical Society of Japan 81 (12), 124008.

Generation of SWAP gate between two remote cavities via an optical fiber by adiabatic passage
L. Molouki, M. Yahyavi, P. Esmaili, E. Talebian
European Physical Journal Plus 127, 134.